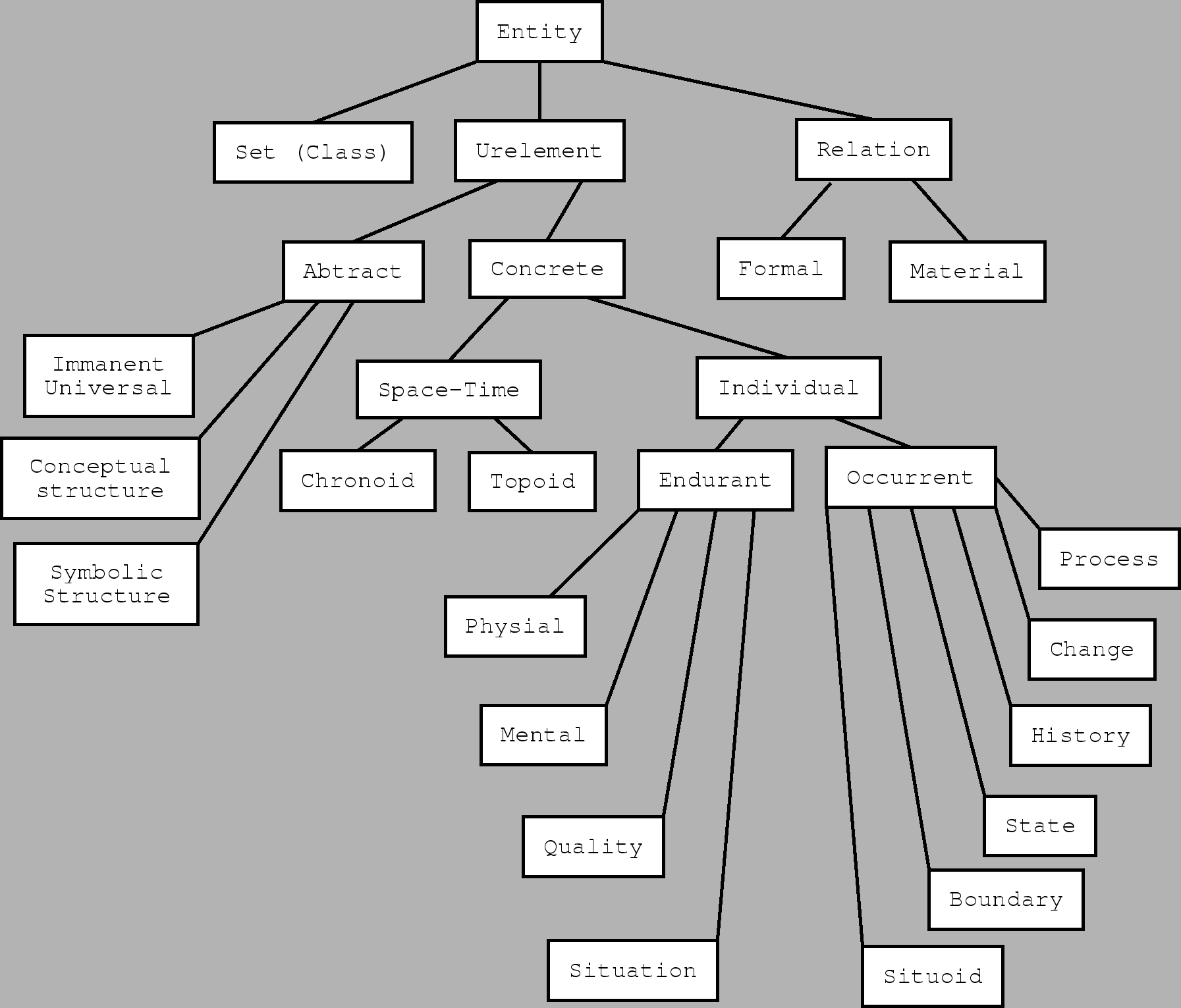
Next: Being part of reality Up: Situoids Previous: Comprehension Contents
Since gestalt theory presupposes a subject capable of comprehension, it is most suited for our theory. We will regard gestalten with situoids as their instances. Gestalt is a German word and refers to the concept where an entities properties cannot be discovered from the total properties of its parts alone The Internet Community. There are similar but different views on the part-whole relationship, the most influential probably being those of phenomenologicians like Husserl soko1,krecz1, who uses the term ``figural moment'' instead of ``gestalt''. More differences between both approaches can be found in gurwitsch.
Alternative theories of the part-of relation, of parts and wholes can be used in an integrated top-level ontology parallel to our theory of whole situoids and their parts, as there may be other categories in the top-level ontology, that do not behave like situoids, as comprehensible wholes.
The philosophy of Brentano, Meinong and Husserl eventually led to the development of gestalt theory reiser1. The founder of the gestalt theoretical movement is Max Wertheimer, although the term ``gestalt theory'' has first been introduced by Christian von Ehrenfels, a disciple of Brentano. Another root of gestalt theory is Ernst Mach becher, whose work ``Beitraege zur Analyse der Empfindung'' (Contributions to the Analysis of Sensations, 1886) influenced gestalt theorists like Ehrenfels, but also phenomenologists like Husserl.
In rescher, three conditions are stated as prerequisites for a whole entity:
All those three conditions have to be regarded concerning a specific part-of relationship, and therefore a specific decomposition.
There are attributes of a whole, that can be shared or unshared by its parts. An attribute, a quality, the whole possesses and none of its parts do is called unshared, and shared if all parts of the whole possess the attribute.
It is clear, that if some attribute is not ![]() -unshared, it does not
necessarily have to be
-unshared, it does not
necessarily have to be ![]() -shared, and vice versa.
-shared, and vice versa.
Underivable attributes of a whole are attributes, that are not a logical consequence of some set of attributes of a set of parts of the whole. Underivable attributes, again, have to be regarded relative to a specific set of parts and attributes. An example of some underivable attribute is the weight of a pile of stones. Of course every part of this pile of stones has a weight, but summoning up the weight of the pile by using the weight of the parts requires the additional natural law that weight is additive. An attribute or quality is derivable if it is a logical consequence of the attributes of some set of parts. Rescher and Oppenheim gave the following definition in rescher
As mentioned, the weight of a pile of stones is not derivable by
purely logical means from the weight of the stones alone. However, we
could add a theory of some kind, and derive this attribute with the help
of this theory. We will call this attribute then
![]() -
-![]() -
-![]() -derivable, if
-derivable, if ![]() is the theory concerned, or
is the theory concerned, or
![]() -
-![]() -
-![]() -underivable, if it is impossible to deduce the attribute
of the whole with the means of
-underivable, if it is impossible to deduce the attribute
of the whole with the means of ![]() . We will call
. We will call
![]() -
-![]() -
-![]() -underivable attributes simply ``underivable''
attributes.
-underivable attributes simply ``underivable''
attributes.
The existence of an underivable attribute corresponds to the First Ehrenfelscriterion, that has been formulated in Christian von Ehrenfels' first study of Gestalt-theory, ``Ueber Gestaltqualitaten'': ``The whole is more than the sum of its parts.'' Sometimes those attributes, that only come into existence when the parts are assembled, are called emergent attributes.
Considering the second condition for wholes, the dependence of certain
characteristics of one part upon those of other parts, we will have
to consider configurations. ``An ordered set of objects,
![]() , which stand in the relation
, which stand in the relation ![]() to each other, i.e. for
which
to each other, i.e. for
which
![]() holds, will be said to form a
configuration of kind
holds, will be said to form a
configuration of kind ![]() .''rescher As we can see,
Rescher and Oppenheim's configurations are certain kinds of states of
affairs, in our terminology, and the kind of the configuration, the
kind of the state of affairs is defined by the relation universal.
.''rescher As we can see,
Rescher and Oppenheim's configurations are certain kinds of states of
affairs, in our terminology, and the kind of the configuration, the
kind of the state of affairs is defined by the relation universal.
For
the easier part of quantitative
attributes, an attribute ![]() of a part
of a part ![]() is called
is called
![]() -dependant upon some class
-dependant upon some class ![]() of quantitative attributes of the
objects
of quantitative attributes of the
objects ![]() , if the
, if the ![]() -value of
-value of ![]() in every configuration of
kind
in every configuration of
kind ![]() is related by
is related by ![]() to the values of the attributes in
to the values of the attributes in ![]() of
of ![]() .
. ![]() may be strong or weak, defining the value of
may be strong or weak, defining the value of ![]() completely, or just statistically. It is possible for the
configuration to consist only of one object, or the class
completely, or just statistically. It is possible for the
configuration to consist only of one object, or the class ![]() consisting only of the attribute
consisting only of the attribute ![]() .
.
For non-quantitative attributes, similar observations can be made. The perceived impression may depend on other parts surrounding or the whole, as can be seen in figure 5.5.
For the requirement that the parts of the whole must stand in some special, characteristic relation of dependence with one another, wholes may form dependence systems, that are defined as follows in rescher:
The third criterion refers to some kind of structure the whole must possess in virtue of its status as a whole. This involves three things:
Often, we are not interested in the specific parts of some whole, but only the types of the parts and the position they occupy in the whole. This leads us to the concept of a complex:
 , constituting the domain
of positions.
, constituting the domain
of positions.
 -position.
-position.
Different complexes may have the same structure, they are
isomorphic. For two complexes to be isomorphic, their topological
domains must have the same structure, the sets of attributes of the
two complexes must have the same structure and the attributes of both
complexes have to be assigned to corresponding positions of the
domains![]() .
.
Isomorphic complexes are related by transformations. Different but isomorphic complexes possess certain structural similarities. Consider a piece of music and another, different piece of music, that is a transposition of the first. They are certainly different, but isomorphic, and they share the same melody. Therefore, there are attributes that are shared by all complexes of a group of isomorphic complexes. They are called complexial features, attributes that are invariant under isomorphic transformations. As Rescher and Oppenheim point out, those attributes may even be underivable. The possession of attributes that are invariant under those transformations is stated as the Second Ehrenfels-criterion.
Let us now apply this formalism to situoids. First we examine what kinds of decompositions of situoids exist. We will examine some possible decompositions shortly, without much discussion and motivation, before we concentrate on one specific decomposition which we will examine further.
Since situoids
exist in time and space, our first possible decomposition is a
spatio-temporal one. We will denote this part-of relation
with
 . For this we project the situoid on parts of its
framing chronoid or topoid or both
. For this we project the situoid on parts of its
framing chronoid or topoid or both![]() .
The attributes we may consider are the
duration or spatial size the resulting entities have. Then we do have
a derivable attribute, namely the new duration and spatial extent the whole
situoid has.
The spatio-temporal parts of the situoid, possibly, but
not necessarily, situoids on its own, may be cause and result of each
other, however, this does not always have to be the case, especially
if the spatio-temporal parts are not situoids.
.
The attributes we may consider are the
duration or spatial size the resulting entities have. Then we do have
a derivable attribute, namely the new duration and spatial extent the whole
situoid has.
The spatio-temporal parts of the situoid, possibly, but
not necessarily, situoids on its own, may be cause and result of each
other, however, this does not always have to be the case, especially
if the spatio-temporal parts are not situoids.
We could also decompose one situoid in multiple sub-situoids, all of
which are part of one situoid. We call this a situoidal
decomposition and denote the appropriate part-of relation
![]() . The parts form a web of situoids, influencing each
other and being cause or result of each other. However, the structure
of the whole situoid is generally derivable by the structure of its
subsituoids.
. The parts form a web of situoids, influencing each
other and being cause or result of each other. However, the structure
of the whole situoid is generally derivable by the structure of its
subsituoids.
Because situoids are described by infons, we can consider an
infonic decomposition of situoids, formed by the relation
![]() or simply
or simply ![]() . The entities that are part of a
situoid in this sense
behave much like situoids and sometimes are situoids, but they contain
less information. They may provide a more abstract view on a situoid,
or a more limited. Consider a situoid
. The entities that are part of a
situoid in this sense
behave much like situoids and sometimes are situoids, but they contain
less information. They may provide a more abstract view on a situoid,
or a more limited. Consider a situoid ![]() of a specific plaza. Then
the infonic parts of this situoid may contain a single human, a
fountain, a tree, one square meter of concrete plate, and so on. When
all those parts are assembled, the situoid
of a specific plaza. Then
the infonic parts of this situoid may contain a single human, a
fountain, a tree, one square meter of concrete plate, and so on. When
all those parts are assembled, the situoid ![]() is an instance of the
universal
is an instance of the
universal ![]() , but none of its parts are. The attribute of ``being
a plaza'' emerged, when the parts where put together. The second
condition however will fail. There is no interdependence between
infons in a situoid. Each infon describes some basic information of a
situoid by stating a relation, a configuration of objects. They may be
informational equivalent, or one is entailing another (logically or
with respect to some theory), but many infons obtaining in situoids
are independent of each other, and are describing different aspects of
the situoid.
, but none of its parts are. The attribute of ``being
a plaza'' emerged, when the parts where put together. The second
condition however will fail. There is no interdependence between
infons in a situoid. Each infon describes some basic information of a
situoid by stating a relation, a configuration of objects. They may be
informational equivalent, or one is entailing another (logically or
with respect to some theory), but many infons obtaining in situoids
are independent of each other, and are describing different aspects of
the situoid.
We have not discussed all the possible decompositions in detail,
as we just wanted to get the idea. There is another decomposition that
we will discuss in more detail,
namely decomposing the situoid
into situations by using the relation
![]() , entities that
exist at time points. This can be
achieved by projecting the situoid on the boundaries of its framing
chronoid. As a result, we get a sequence of situations. We will now
examine this decomposition in detail and try to find out, whether
situoids fulfil all three conditions of wholes regarding this
decomposition.
, entities that
exist at time points. This can be
achieved by projecting the situoid on the boundaries of its framing
chronoid. As a result, we get a sequence of situations. We will now
examine this decomposition in detail and try to find out, whether
situoids fulfil all three conditions of wholes regarding this
decomposition.
First we have to examine whether the relation
![]() defines a
decomposition of situoids. A situation is the projection of a situoid
on one of the time boundaries of its framing chronoid. Therefore,
defines a
decomposition of situoids. A situation is the projection of a situoid
on one of the time boundaries of its framing chronoid. Therefore,
![]() , iff
, iff ![]() is a situation,
is a situation, ![]() a situoid,
a situoid, ![]() the
chronoid framing
the
chronoid framing ![]() and
and ![]() is
the projection of
is
the projection of ![]() on one of the time boundaries of
on one of the time boundaries of ![]() . Let
. Let ![]() be the
class of all situations of the situoid
be the
class of all situations of the situoid ![]() . We can see, that
. We can see, that
![]() is not reflexive, so we will consider it an
irreflexive part-of relation.
Then,
is not reflexive, so we will consider it an
irreflexive part-of relation.
Then, ![]() is a
decomposition of
is a
decomposition of ![]() regarding the part-of relation
regarding the part-of relation
![]() if
every
if
every
![]() -part of
-part of ![]() overlaps with some
overlaps with some
![]() -part of
-part of ![]() . However, our understanding of the
relation
. However, our understanding of the
relation
![]() defies the usual understanding of a part-of
relation, as it is a relation between two distinct classes of
entities, situations and situoids, and cannot be applied to the
parts. For the sake of the argument, we will make the relation
defies the usual understanding of a part-of
relation, as it is a relation between two distinct classes of
entities, situations and situoids, and cannot be applied to the
parts. For the sake of the argument, we will make the relation
![]() reflexive:
reflexive:
![]() .
.
Now we can show, that ![]() , the class
of all situations defined by a situoid
, the class
of all situations defined by a situoid ![]() , is a
decomposition of
, is a
decomposition of ![]() .
Let
.
Let
![]() . Then it can be easily shown, that
. Then it can be easily shown, that
![]() , because
, because ![]() contains all the situational parts of
contains all the situational parts of
![]() .
.
The result is pretty trivial, but will become more useful when we
start extending the relation
![]() . The class
. The class ![]() of all
situations of the situoid
of all
situations of the situoid ![]() is still a decomposition of the relation
is still a decomposition of the relation
![]() . We can also extend this relation
further, by adding an infonic part-of relation on situations, and
. We can also extend this relation
further, by adding an infonic part-of relation on situations, and
![]() will still be a decomposition of
will still be a decomposition of ![]() .
.
Obviously, the sum of all situations in chronological order is a sequence of entities, that can be described by states of affairs, just like in situation theory, or situation calculus. Our second condition for wholes is satisfied, because situations may be causes of their following situations, or results of previous situations. Therefore, the infons obtaining in one situation may depend on the infons obtaining in previous situations and with this, the perception of one situation may depend on their neighboring situations and the whole.
Let us consider an example to illustrate this idea. Imagine a 100
meter race as a situoid. Two people are running, ![]() and
and ![]() . So we
will have a situoid
. So we
will have a situoid ![]() with a topoid
with a topoid ![]() and
and ![]() . The runners
start at the location
. The runners
start at the location ![]() and finish at
and finish at
![]() . The race
lasts 20 seconds, so there is a chronoid
. The race
lasts 20 seconds, so there is a chronoid ![]() with
with
![]() .
. ![]() needs 10 seconds to reach the finish line,
therefore running during the chronoid
needs 10 seconds to reach the finish line,
therefore running during the chronoid ![]() ,
, ![]() needs 20
seconds. We will express this with the timed
infons
needs 20
seconds. We will express this with the timed
infons
![]() and
and
![]() and
and
![]() and
and
![]() . Projecting
. Projecting ![]() on the
right boundary of
on the
right boundary of ![]() we will obtain a situation
we will obtain a situation ![]() where
where ![]() is
finishing the entire race,
is
finishing the entire race, ![]() :
:
![]() . The second situation will be the projection of
. The second situation will be the projection of ![]() on the right boundary of
on the right boundary of ![]() ,
, ![]() . A similar infon obtains here,
. A similar infon obtains here,
![]() , but due to the position of
, but due to the position of ![]() in
in ![]() we know, that he did not win the race:
we know, that he did not win the race:
![]() . However, a detailed investigation of causality in the
framework of GOL, and therefore also in the framework of situoids and
situations is still an open research topic.
. However, a detailed investigation of causality in the
framework of GOL, and therefore also in the framework of situoids and
situations is still an open research topic.
The situoid is structured into situations as the chronoid is in time boundaries, because we are only projecting the situoid on its framing chronoids time boundaries. For the third condition - that the situoid must possess some kind of structure, in virtue of which certain structural characteristics pertain to it - to be satisfied we will need to identify the parts, a domain of positions the parts may occupy and an assignment function assigning each part to a position. The parts are the situations, the positions the time boundaries and the assignment function is our projection of the situoid on the time boundaries. As Rescher and Oppenheim point out in rescher, we usually do not care about the individual parts, but rather about the types of the parts. Doing so, they call the entity of concern a ``complex'', while we will talk about ``universal'' in a more general approach.
We assumed that every situoid is an instance of some universal. Universals define the structure whose possession is required of wholes in rescher. Universals may require the existence of certain situations in a situoid. These are the parts that are assigned to spatio-temporal locations inside the situoid by the universal. Then, a ``transposition'' in the sense of rescher is nothing more than another instance of the same universal. And usually, there are features of a situoid which are solely due to its being an instance of a specific universal.
For example, the universal ``100 meter race'' will require the
existence of certain situations, at least a starting and an ending
situation, which are assigned to the left respectively the right
boundary of the chronoid framing the situoid ![]() . The other parts
(situations) required by an universal which
. The other parts
(situations) required by an universal which ![]() is an instance of, if
any, behave as well and are accordingly assigned to certain
spatiotemporal locations. It is this structure - the assignment of
situations to proper space-time locations - which makes some situoid
a 100 meter race
is an instance of, if
any, behave as well and are accordingly assigned to certain
spatiotemporal locations. It is this structure - the assignment of
situations to proper space-time locations - which makes some situoid
a 100 meter race![]() and which makes it an instance of the universal ``100 meter
race''.
and which makes it an instance of the universal ``100 meter
race''.
Now the question is, whether a situoid is more than just a mere sequence of situations. This is the case, because situoids are continuous. Just like chronoids cannot be understood as sets of time boundaries, but are inherently more, situoids are inherently more than collections of situations. There are even processes, that can never be fully understood in the domain of situations, but only in a continuous structure like situoids.
Let us consider an example, illustrated in figure
5.6![]() . There are
two people,
. There are
two people, ![]() and
and ![]() 100 meter
apart. Both are walking towards each other in a straight line with a
velocity of one
meter per second. A bird flies between
100 meter
apart. Both are walking towards each other in a straight line with a
velocity of one
meter per second. A bird flies between ![]() and
and ![]() , starting from
, starting from ![]() ,
with 100 meters per second. When the bird reaches
,
with 100 meters per second. When the bird reaches ![]() or
or ![]() , it turns
around in no time, and flies the other way. There are three concurrent
processes, one depending on two others. These processes can be
embedded in a situoid, but cannot be understood in the domain of
situations. In the domain of situations this example would be modelled
by a sequence of situations, at least one for every occurring
change. So whenever the bird reaches
, it turns
around in no time, and flies the other way. There are three concurrent
processes, one depending on two others. These processes can be
embedded in a situoid, but cannot be understood in the domain of
situations. In the domain of situations this example would be modelled
by a sequence of situations, at least one for every occurring
change. So whenever the bird reaches ![]() or
or ![]() , a situation is needed
to state that the bird is changing direction. But when we would ask
which direction the bird would be facing when
, a situation is needed
to state that the bird is changing direction. But when we would ask
which direction the bird would be facing when ![]() and
and ![]() meet, we
cannot get an answer if these processes are modelled as sequences of
situations. We will not get an answer either in the domain of
situoids, but there we cannot ask the question. There are just three
processes, one depending on two others, and the changes of heading are
of no concern.
meet, we
cannot get an answer if these processes are modelled as sequences of
situations. We will not get an answer either in the domain of
situoids, but there we cannot ask the question. There are just three
processes, one depending on two others, and the changes of heading are
of no concern.
There are more Zeno-like paradoxa which can be used to argue in favor
of situoids being more than a mere sequence of situations. Imagine
Zenos arrow, which is not moving at any point in time, but still
changing position. In the domain of situoids, this arrow is indeed
moving in a straight line with a velocity ![]() , while in the domain of
situations this arrow may be changing positions while its velocity is
zero. However, more research has to be done with respect to examples
such as the above flying-bird example, as the explanation of why it
is impossible to determine the direction in which the bird is flying
at the end is somewhat awkward.
, while in the domain of
situations this arrow may be changing positions while its velocity is
zero. However, more research has to be done with respect to examples
such as the above flying-bird example, as the explanation of why it
is impossible to determine the direction in which the bird is flying
at the end is somewhat awkward.
But still we believe the first condition for wholes satisfied. The
whole situoid is more than the sum of its
![]() -parts.
-parts.
We have found a decomposition and discussed a set of attributes, that
fulfills all our conditions for wholes, and can therefore regard
situoids as ``whole'' at least with regard to the
![]() relation.
relation.
leechuck 2005-04-19